Question

A cyclist is traveling at 10m/s when he comes to a hill. He stops pedaling at the bottom of the hill and lets the bicycle coast up the hill. Assuming no energy is lost to friction and ???? equals 10m/s2 , what will be the vertical height of the bicycle when it stops coasting?

Answer 1

Vertical height = 5 m

Step-by-step explanation:

Given:

There is no frictional losses. So, energy is conserved.

Acceleration due to gravity (g) = 10 m/s²

Initial velocity at the bottom of hill (u) = 10 m/s

Final velocity at the moment it stops on the hill (v) = 0 m/s

Initial height at the bottom (h₁) = 0 m

Final height (h₂) = ?

As there are no frictional losses, the total energy remains conserved.

So, increase in potential energy is equal to decrease in kinetic energy.

Increase in potential energy is given as:

`\Delta U=mg(h_2-h_1)=10mh_2`

Decrease in kinetic energy is given as:

`\Delta KE=(1)/(2)m(u^2-v^2)=(1)/(2)m(10^2)=50m`

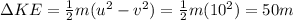
Now,
`\Delta U =\Delta KE`

⇒
`10mh_2=50m`

⇒
`h_2=(50)/(10)=5\ m`

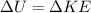
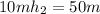
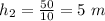
Therefore, the vertical height of the bicycle when it stops coasting is 5 m.