Question

What is the area of the composite figure whose vertices have the following coordinates?

(−2, −2) , (4, −2) , (5, 1) , (2, 3) , (−1, 1)

Answer 1

24 square units

Explanation:

The area of a polygon can be computed from its list of coordinates as ...

`\displaystyle A=(1)/(2)\left|\sum\limits_(k=1)^n{x_k(y_(k+1)-y_(k-1))}\right|`

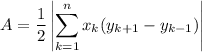
where there are n coordinate points. Subscripts on y "wrap around" so that ...

`y_(n+1)=y_1\\y_0=y_n`

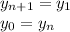
The area from these coordinates is ...

A = (1/2)|-2(-2-1) +4(1-(-2)) +5(3-(-2)) +2(1-1) -1(-2-3)|

= (1/2)|6 +12 +25 +0 +5|

= (1/2)(48)

= 24 . . . . square units

_____

If you graph the points, you see that the figure can be considered to be a parallelogram topped by a triangle. The area of the parallelogram is ...

A = bh = 6·3 = 18

The area of the triangle is ...

A = 1/2bh = (1/2)(6)(2) = 6

Then the total area is 18+6 = 24, which agrees with the above calculation.

Answer 2

24

Explanation:

From the picture you can see that area is sume of area P1 and area P2.

a=6 because its egdes are on point -2 and 4, so it is 6. (See x-ose)

h=3 Edges on -2 and 1 (see y-ose)

k=2 edges on 3 and 1 on y-ose

P1=a*h=6*3=18

P2=a*k/2=6*2/2=6

P=18+6=24