Answer:
60 inches long are the sides of the pillars.
Explanation:
Given : A small bridge sits atop four cube shaped pillars that all have the same volume. the combined volume of the four pillars is 500 ft cubed.
To find : How many inches long are the sides of the pillars?
Solution :
Refer the attached picture below for Clarence of question.
The volume of the cube is

Where, a is the side.
The combined volume of the four pillars is 500 ft cubed.
The volume of each cube is given by,
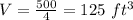
Substitute in the formula to get the side,
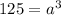
![a=\sqrt[3]{125}](https://img.qammunity.org/2021/formulas/mathematics/high-school/msl5a1ydw660vececkvjbvn6ravigtojza.png)
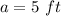
We know, 1 feet = 12 inches
So, 5 feet =
 inches
inches
Therefore, 60 inches long are the sides of the pillars.