Question

The area of parallelogram is 104 square units and base length is 13. What is the height of the parallelogram?

Answer 1

• 8 units

⠀

Explanation :

⠀

Here,

• Area of the parallelogram is 104 sq. units

• Base length of the parallelogram is 13 units.

⠀

We know that,

`{\longrightarrow \qquad{ \mathfrak{ \pmb{Base * Height = Area_((Parallelogram))}}}}`

⠀

Now, Substituting the values in the formula :

⠀

`{\longrightarrow \qquad{ \sf{ {13 * Height = 104}}}}`

⠀

`{\longrightarrow \qquad{ \sf{ {Height = (104)/(13) }}}}</p><p>`

⠀

`{\longrightarrow \qquad{ \pmb { \frak{Height \: \: {= 8}}}}}`

⠀

Therefore,

• The Height of the parallelogram is 8 units.

Answer 2

Question :-

• The Area of Parallelogram is 104 units² . Its Base is 13 units . What is the Height of the Parallelogram ?

Answer :-

• Height of Parallelogram is 8 units .

Explanation :-

As per the provided information in the given question, we have been given that the Area of Parallelogram is 104 units² . Its Base is given as 13 units . And, we have been asked to calculate the Height of the Parallelogram .

For calculating the Height , we will use the Formula :-

`\bigstar \: \: \: \boxed{ \sf{ \: Area \: _(Parallelogram) \: = \: Base \: * \: Height \: }}`

Therefore , by Substituting the given values in the above Formula :-

`\dag \: \: \: \sf { Area \: _(Parallelogram) \: = \: Base \: * \: Height }`

`\longmapsto \: \: \: \sf { 104 \: = \: 13 \: * \: Height }`

`\longmapsto \: \: \: \sf { \frac {104}{13} \: = \: Height}`

`\longmapsto \: \: \: \sf { 8 \: = \: Height}`

`\longmapsto \: \: \: \textbf {\textsf {Height \: = \: 8 }}`

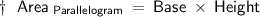
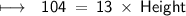
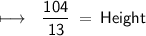
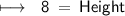
Hence :-

• Height of Parallelogram = 8 units .

`\underline {\rule {204pt} {4pt}}`

Additional Information :-

`\begin{gathered} \begin{gathered} \begin{gathered}\begin{gathered}\boxed{\begin{array}{c} \\ \underline{ { \textbf {\textsf \red{ \dag \: \: More \: Formulas \: \: \dag}}}} \\ \\ \\ \footnotesize \bigstar \: \bf{Area \: _(Square) = Side * Side} \\ \\ \\ \footnotesize\bigstar \: \bf{Area \: _(Rectangle) = Lenght * Breadth} \\ \\ \\ \footnotesize \bigstar \: \bf{Area \: _(Triangle) = (1)/(2) * Base * Height } \\ \\ \\ \footnotesize \bigstar \: \bf{Area \: _(Parallelogram) = Base * Height} \\ \\ \\ \footnotesize \bigstar \: \bf{Area \: _(Trapezium) = (1)/(2) * [ \: A + B \: ] * Height } \\ \\ \\ \footnotesize \bigstar \: \bf {Area \: _(Rhombus) = (1)/(2) * Diagonal \: 1 * Diagonal \: 2}\end{array}}\end{gathered}\end{gathered} \end{gathered} \end{gathered}`