Question

PLEASE HELP I AM TRYING TO STUDY FOR A CALCULUS EXAM!! WORTH A TOTAL OF 100 POINTS!!!!!!!!!

Find the mass of a sphere of radius 7 if δ= kρ, k a constant.

Answer 1

Presumably, both δ and ρ represent density of some object. Considering the context, ρ is likely a function of 3 variables, ρ = ρ(x, y, z). Then the mass of the sphere with the prescribed density δ(x, y, z) = k ρ(x, y, z) is

`\displaystyle \iiint_B k \rho(x,y,z) \, dx\, dy\, dz`

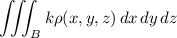
where B is the set

`B = \left\{(x,y,z) ~ : ~ x^2+y^2+z^2 \le 7^2\right\}`

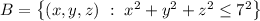
If you have all the details at hand, you can compute the integral by converting to spherical coordinates, substituting

`\begin{cases}x = u \cos(v) \sin(w) \\ y = u \sin(v) \sin(w) \\ z = u \cos(w)\end{cases} \text{ and } dx\,dy\,dz = u^2 \sin(w) \, du \, dv \, dw`

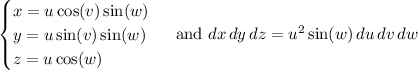
The integral then transforms to

`\displaystyle k \int_0^(2\pi) \int_0^\pi \int_0^7 \rho(u\cos(v)\sin(w),u\sin(v)\sin(w),u\cos(w)) \, u^2 \sin(w) \, du \, dw \, dv`

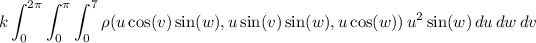
Without any additional information, there's not much more to say...