Answer:
Step-by-step explanation:
mass of alpha particle, m = 4 u = 4 x 1.67 x 10^-27 kg
charge, q = 2 e = 3.2 x 10^-19 C
radius, r = 3.45 cm
Magnetic field, B = 1.67 T
(a) let v be the speed
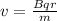
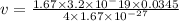
v = 2760000 m/s
(b) Let T be the period of revolution

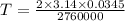
T = 7.85 x 10^-8 seconds
(c) Kinetic energy, K =0.5 x mv²
K = 0.5 x 4 x 1.67 x 10^-27 x 2760000 x 2760000
K = 6.36 x 10^-15 J
(d) kinetic energy, K = e V
where, V is the potential difference
6.36 x 10^-15 = 1.6 x 10^-19 x V
V = 39754.35 V