Question

A rectangular wall is covered entirely with two kinds of decorative tiles: regular and jumbo. 1/3 of the tiles are jumbo tiles, which have a length three times that of regular tiles and have the same ratio of length to width as the regular tiles. If regular tiles cover 80 square feet of the wall, and no tiles overlap, what is the area of the entire wall?

Answer 1

The total area of the entire wall = 440 sq feet.

Explanation:

Here, let us assume:

The total number of tiles = T

The total number of jumbo tiles = J

The total number of regular tiles = J

⇒ R + J = T or, R + J = 3 J

⇒ R = 3J - J

⇒ R = 2 J

⇒ J =
`((R)/(2))`

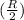
Now, let us assume the length of regular tile = L

And the width of the regular tile = W

So, the area of 1 regular tile = Length x Width = L x W =
`A_r`

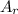
So, the length of the jumbo tile = 3 x ( Length of Regular tile ) = 3 L

⇒The width of the jumbo tile = 3 x ( width of Regular tile ) = 3 W

So, the area of 1 jumbo tile = Length x Width = (3 L) x (3 W) =
`A_j`

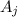
= 9 L W = 9 (Area of 1 regular tile) = 9
`A_r`

⇒
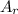
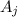`A_j` = 9
`A_r`

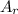
Now, the total area of regular tiles = 80 sq ft

So, Number of regular tiles x Area of 1 regular tile = 80 sq ft

Now, the total area A = Number of jumbo tiles x Area of each jumbo tile + Number of regular tiles x Area of each regular tile

`A = R A_r + J A_j\\= R A_r + (R)/(2) (9A_r) = (11)/(2) RA_r\\= (11)/(2) * 80 = 440\\\implies A = 440`

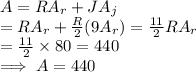
Hence, the total area of the entire wall = 440 sq feet.