Question

A light train made up of two cars is traveling at 90 km/h when the brakes are applied to both cars. Know that car A has a mass of 31 Mg and car B has a mass of 20 Mg, and the braking force is 41 kN on each car. Determine:

(a) the distance traveledby the train before it comes to a stop
(b) the coupling force between the cars as the is slowing down.

Answer 1

a)
`d=236.280\,m`, b)
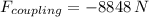`F_(coupling) = -8848\,N` The real force has the opposite direction.

Step-by-step explanation:

a) Let assume that train moves on the horizontal ground. An equation for the distance travelled by the train is modelled after the Principle of Energy Conservation and Work-Energy Theorem:

`K_(A) = W_(brake)`

`(1)/(2)\cdot m_(train) \cdot v^(2) = F_(brakes)\cdot d`

`d = (m_(train)\cdot v^(2))/(2\cdot F_(brakes))`

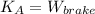
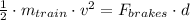
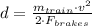
`d = ((51000\,kg)\cdot [(90\,(km)/(h) )\cdot ((1000\,m)/(1\,km) )\cdot ((1\,h)/(3600\,s) )]^(2))/(2\cdot (82000\,N))`

`d=194.360\,m`

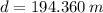
b) The acceleration experimented by both trains are:

`a = -(v_(o)^(2))/(2\cdot d)`

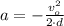
`a = -([(90\,(km)/(h) )\cdot ((1000\,m)/(1\,km) )\cdot ((1\,h)/(3600\,s))]^(2))/(2\cdot (194.360\,m))`

`a = -1.608\,(m)/(s^(2))`

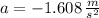
The coupling force in the car A can derived of the following equation of equilibrium:

`\Sigma F = F_(coupling) - F_(brakes) = m_(A)\cdot a`

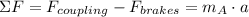
The coupling force between cars is:

`F_(coupling) = m_(A)\cdot a + F_(brakes)`

`F_(coupling) = (31000\,kg)\cdot(-1.608\,(m)/(s^(2)) )+41000\,N`

`F_(coupling) = -8848\,N`

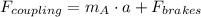
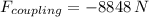
The real force has the opposite direction.