Question

Students conducted a survey and found out that 36% of their peers on campus had tattoos but only 4% of their peers were smokers. If 100 students were surveyed, can these students use the Normal approximation to construct a confidence interval for the proportion of students in the population who are smokers? Yes, because both n p and n ( 1 − p ) are less than 15. Yes, because both n p and n ( 1 − p ) are greater than 15. No, because either n p or n ( 1 − p ) are less than 15. No, because either n p or n ( 1 − p ) are greater than 15.

Answer 1

No, because either np or n(1 − p) are less than 15.

Explanation:

If a random variable X follows a Binomial distribution with parameters n and p and if n is large and p is small then the Normal approximation can be used to approximate the distribution of sample proportion
`\hat p`.

The conditions to be applied for Normal approximation are:

• np ≥ 15
• n(1 - p) ≥ 15

The random variable X can be defined as the number of students who are smokers.

Given:

n = 100

p = 0.04

Compute the value of np and n(1 - p) as follows:

`np=100*0.04=4\\n(1-p)=100* (1-0.04)=96`

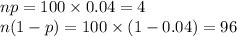
So n(1 - p) > 15 but np < 15.

Hence, the Normal approximation cannot be used to construct a confidence interval for the proportion of students in the population who are smokers.

The correct option is: No, because either np or n(1 − p) are less than 15.