Question

A 0.200 kg potato is tied to a string with length 2.10 m , and the other end of the string is tied to a rigid support. The potato is held straight out horizontally from the point of support, with the string pulled taut, and is then released.

a. What is the speed of the potato at the lowest point of its motion? let g be = 9.80 m/s^2b. What is the tension on the string at this point?

Answer 1

To solve this problem we will apply the concepts related to the balance of energy and forces. For the first part we will equalize the potential energy and the kinetic energy with which we can find the speed of the body. Later we will use the balance of forces that put the body in equilibrium to find the tension of the spring.

Our values are defined as,

m = 0.2 kg

r = 2.1 m

PART A) Equating potential energy at the start to kinetic energy at the lowest point:

`mgr = (mv^2)/(2)`

`v^2 = 2gr`

`v = √(2gr)`

`v = √(2*9.8*2.1)`

`v= 6.41 m/s`

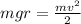
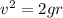
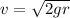
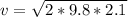
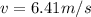
Therefore the velocity of the potato at the lowest point of its motion is 6.41m/s

PART B) Now, equating the forces to get the tension required:

`T - mg = (mv^2)/(r)`

`T = mg + ((v^2)/(r))}`

`T = 0.2*9.8 + (6.41^2)/(2.1)`

`T= 21.52 N`

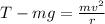
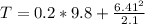
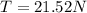
Therefore the Tension on the spring at this point is 21.52N