Question

Tom determines that the system of equations below has two solutions, one of which is located at the vertex of the parabola.

Equation 1: (x – 3)2 = y – 4
Equation 2: y = -x + b
In order for Tom’s thinking to be correct, which qualifications must be met?

Answer 1

Answer: A is correct

Step-by-step explanation: b must equal 7 and a second solution to the system must be located at the point (2, 5).

Answer 2

Option A: b must equal 7 and a second solution to the system must be located at the point (2, 5)

Explanation:

The complete question is

Tom determines that the system of equations below has two solutions, one of which is located at the vertex of the parabola.

Equation 1: (x – 3)2 = y – 4

Equation 2: y = -x + b

In order for Tom’s thinking to be correct, which qualifications must be met?

A: b must equal 7 and a second solution to the system must be located at the point (2, 5).

B: b must equal 1 and a second solution to the system must be located at the point (4, 5).

C: b must equal 7 and a second solution to the system must be located at the point (1, 8).

D: b must equal 1 and a second solution to the system must be located at the point (3, 4).

step 1

Find the vertex of the quadratic equation

The general equation of a vertical parabola in vertex form is

`y=a(x-h)^2+k`

where

(h,k) is the vertex

we have

`(x-3)^(2)=y-4`

so

`y=(x-3)^(2)+4`

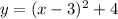
The vertex is the point (3,4)

step 2

Find out the value of b in the linear equation

we know that

If the vertex is a solution of the system of equations, then the vertex must satisfy both equations

substitute the value of x and the value of y of the vertex in the linear equation

`y=-x+b`

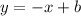
For x=3, y=4

`4=-3+b\\b=7`

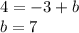
so

`y=-x+7`

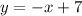
step 3

Find out the second solution of the system of equations

we have

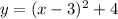`y=(x-3)^(2)+4` -----> equation A

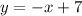`y=-x+7` ----> equation B

solve the system of equations by graphing

Remember that the solutions are the intersection points both graphs

The second solution of the system of equations is (2,5)

see the attached figure

therefore

b must equal 7 and a second solution to the system must be located at the point (2, 5)