Question

Perry has an opportunity to invest with an APR of 5.25%.

Using the rule of 70, how long will it take his investment to double? (Round to the hundredths.)




If you had a bank that offered 6.1% interest and compounded money 4 times a year, how many years would go by until you could turn $6000 into enough to buy a brand new car ($12,000)?



For this problem, you will want to guess and check until you find the right solution.

How many years does it take for $100 to reach $1 million dollars? The rate is at 5% with it being compounded twice a year. Round to a whole year. Hint: You may want to start with numbers above 100 years!

Answer 1

1. 13.33 years

2. 11 years

3. 186 years

Step-by-step explanation:

Problem 1. Perry has an opportunity to invest with an APR of 5.25%.

Using the rule of 70, how long will it take his investment to double? (Round to the hundredths.)

Solution

The rule of 70 permits to make a quick estimation of the number of years an investment would double its value, depending on the annual percentage rate (APR).

The formula used for the rule of 70 is:

`\text{Number of Years to Double}=\frac{70}{\text{APR}}`

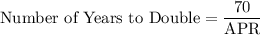
Substitute with APR = 5.25 and compute:

`\text{Number of Years to Double}=\frac{70}{\text{0.0525}}=13.33years`

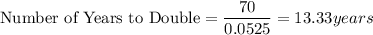
Problem 2. If you had a bank that offered 6.1% interest and compounded money 4 times a year, how many years would go by until you could turn $6000 into enough to buy a brand new car ($12,000)?

For this problem, you will want to guess and check until you find the right solution.

Solution

You have to use the formula for monthly compound interest:

`A=P* \bigg(1+(r)/(n)\bigg)^((n* t))`

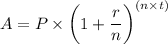
Where:

• A is the the value after adding the interests: $12,000
• P is the value invested: $6,000
• r is the APR: 6.1% = 0.061
• n is the number of times the interest is compounded per year: 4
• t is the number of years: your unknown

Substitute:

`\$12,000=\$6,000* \bigg(1+(0.061)/(4)\bigg)^((4* t))\\\\\\2=1.01525^(4t)`

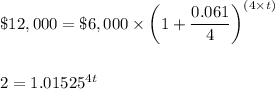
Guess and check until you find the right solution:

Your first educated guess may be using the rule of 70.

• Number of years = 70/6.1 = 11.48

Use t = 12 ⇒ 4t = 4(12) = 48

• 1.01525⁴⁸ = 2.067 . . . pretty close to 2

Use t = 11 to verify which is closer to 2:

• 4t = 4(11) = 44

• 1.01525⁴⁴ = 1.946

t = 11 is closer because 2 - 1.946 = 0.054 and 2.067 - 2 = 0.067

Hence, the best calculation is 11 years.

Problem 3. How many years does it take for $100 to reach $1 million dollars? The rate is at 5% with it being compounded twice a year. Round to a whole year. Hint: You may want to start with numbers above 100 years!

Solution

Use the same formula for monthly compound interest:

`A=P* \bigg(1+(r)/(n)\bigg)^((n* t))`

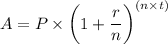
With:

• A: $100
• P: $1,000,000
• r: 5% = 0.05
• n: 2
• t: your unknown

Substitute:

`\$1,000,000=\$100* \bigg(1+(0.05)/(2)\bigg)^((2* t))\\\\\\10,000=1.025^(2t)`

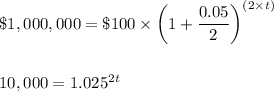
Guess and check until you find the right solution.

Start with t = 100 years:

• 1.025²⁰⁰ ≈ 140 . . . very far from 10,000

Try t = 200 years:

• 1.025⁴⁰⁰ = 19,478

t = 150

• 1.025³⁰⁰ = 1,648

t = 186

• 1.025³⁷² = 9,756

t = 187

• 1.025³⁷⁴ = 10,250

9,756 is closer to 10,000 than 10,250, thus the answer is 186 years.