Question

Suppose we will flip a fair coin 100 times. Let X be the number of heads, and use a Normal distribution to approximate the distribution of X.

a. Apply the continuity correction:

P(42<= X<= 58)= P(_____)
(b) Apply the continuity correction:

P(X > =50) = P( X >_____

Answer 1

(a) P (42 ≤ X ≤ 58) = 0.9108

(b) P (X ≥ 50) = 0.5398

Explanation:

The random variable X is defined as the number of heads.

The probability of tossing a Heads in a single flip is, P (X) = p = 0.50.

The coin was flipped n = 100 times.

The random variable thus follows a Binomial distribution with parameters n and p.

As the sample size is too large (n > 30) and the probability of success is closer to 0.50, the binomial distribution can be approximated by the Normal distribution.

The mean of this distribution is:
`\mu=np=100*0.50=50`.

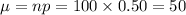The standard deviation of this distribution is:
`\sigma=√(np(1-p))=√(100*0.50*(1-0.50))=5`

(a)

Compute the value of P (42 ≤ X ≤ 58) by applying the continuity correction as follows:

After applying the continuity correction the probability statement is:

`P (42-0.5 \leq X \leq 58+0.5)=P(41.5< X< 58.5)`

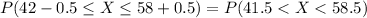
The probability is:

`P(41.5<X< 58.5)=P((41.5-50)/(5)< X< (58.5-50)/(5))\\=P(-1.75<Z<1.7)\\=P(Z<1.7)-P(Z<-1.7)\\=P(Z<1.7)-[1-P(Z<1.7)]\\=2P(Z<1.7)-1\\=(2*0.9554)-1\\=0.9108`

*Use a z-table for the probability value.

Thus, the value of P (42 ≤ X ≤ 58) by applying the continuity correction is 0.9108.

(b)

Compute the value of P (X ≥ 50) by applying the continuity correction as follows:

After applying the continuity correction the probability statement is:

`P(X\geq 50-0.5)=P(X>49.5)`

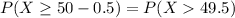
The probability is:

`P(X>49.5)=P((X-\mu)/(\sigma)>(49.5-50)/(5) )\\=P(Z>-0.1)\\=P(Z<0.1)\\=0.5398`

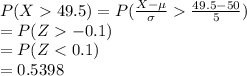
Thus, the value of P (X ≥ 50) by applying the continuity correction is 0.5398.