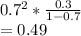Question

The probability of a customer arrival at a grocery service counter in any one second is equal to 0.3. Assume that customers arrive in a random stream, so an arrival in any one second is independent of all others. (Round your answers to four decimal places.) (a) Find the probability that the first arrival will occur during the third one-second interval. (b) Find the probability that the first arrival will not occur until at least the third one-second interval.

Answer 1

The probability that the first arrival will occur during the third one-second interval is 0.51. The probability that the first arrival will not occur until at least the third one-second interval is 0.49.

Step-by-step explanation:

To find the probability that the first arrival will occur during the third one-second interval, we need to find the probability that the first two intervals do not have any arrivals and the third interval has an arrival. Since the probability of a customer arrival in any one second is 0.3, the probability that the first interval does not have any arrival is 0.7, and the probability that the first two intervals do not have any arrivals is 0.7 * 0.7 = 0.49. Thus, the probability that the first arrival will occur during the third interval is 1 - 0.49 = 0.51.

To find the probability that the first arrival will not occur until at least the third one-second interval, we need to find the probability that the first two intervals do not have any arrivals. As calculated before, the probability that the first two intervals do not have any arrivals is 0.49. Thus, the probability that the first arrival will not occur until at least the third interval is 0.49.

Answer 2

Step-by-step explanation:

Given that the probability of a customer arrival at a grocery service counter in any one second is equal to 0.3

Assume that customers arrive in a random stream, so an arrival in any one second is independent of all others.

i.e. X the no of customers arriving is binomial with p = 0.3 and q = 1-0.3 =0.7

a) the probability that the first arrival will occur during the third one-second interval.

= Prob that customer did not arrive in first 2 seconds * prob customer arrive in 3rd sec

=
`0.7^2 (0.3)\\= 0.147`

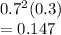
b) the probability that the first arrival will not occur until at least the third one-second interval.

Prob that customer did not arrive in first two seconds *(Prob customer arrives in 3rd or 4th or 5th.....)

=
`(0.7^2)[0.3+0.7*0.3+0.7^2*0.3+....)\\`

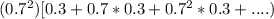
The term inside bracket is a geometric infinite progression with common ratio - 0.7 <1

Hence the series converges

Prob =
`0.7^2 *(0.3)/(1-0.7) \\=0.49`