Answer:
P(X = 7) = 0.3826
Explanation:
We are given that a hotel claims that 90% of its customers are very satisfied with its service. A random sample of eight customers is chosen.
The Binomial probability distribution is given by;

where, n = number of trials (samples) taken = 8
r = number of success
p = proportion of customers that are satisfied with hotel's service,
i.e.; p = 0.90
Let = Number of customers that are very satisfied
So, Probability that seven customers are very satisfied = P(X = 7)
P(X = 7) =
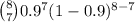
= 8 *
 = 0.3826
= 0.3826
Therefore, probability that seven customers are very satisfied is 0.3826.