Answer:
(a) 0.1326
(b) 0.2732
(c) 0.0410
Explanation:
Let X = number of defective components.
The probability of X is, P (X) = p = 0.02.
The random variable X follows a Binomial distribution with parameters n and p. The probability mass function of a Binomial distribution is:
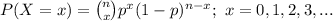
(a)
Compute the probability that the 100 orders can be filled without reordering components as follows:
n = 100
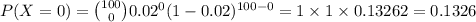
Thus, the probability that the 100 orders can be filled without reordering components is 0.1326.
(b)
Compute the probability that out of 102 orders 2 orders needs reordering as follows:
n = 102
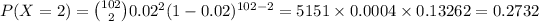
Thus, the probability that out of 102 orders 2 orders needs reordering is 0.2732.
(c)
Compute the probability that out of 105 orders 2 orders needs reordering as follows:
n = 105
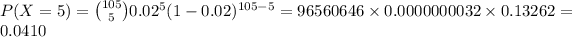
Thus, the probability that out of 105 orders 5 orders needs reordering is 0.0410.