Question

An object is thrown straight up from the top of a 100-foot platform at a velocity of 48 feet per second. The height h(t) of the object t seconds after being thrown is given by
`h(t)=-16t^(2)+48t+100`. Find the maximum height reached by the object and the time it takes to achieve this height.

Answer 1

• The maximum height is 136 ft

• The time it takes to achieve this height is 1.5 s.

Step-by-step explanation:

1. Function for the height (given):

`h(t)=-16t^2+48t+100`

2. Type of function

That is a quadatic function, whose graph is a parabola that opens downward.

The maximum of the function, i.e. the maximum height, is the vertex of the parabola.

The vertex of a parabola with the genral equation
`y=ax^2+bx+c` is at the x-coordinate

`x=-b/(2a)`

3. Time to achieve the maximum height

Substitute b with 48 and a with - 16:

`t=-48/(2(-16))=48/32=3/2=1.5`

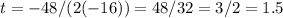
Then, time when the object achieves the maximum height it 1.5s

4. Maximum height:

Replace t with 1.5 in the equation, to find the maximum height, h(1.5)

`h(1.5)=-16(1.5)^2+48(1.5)+100=136`

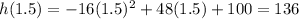
Then, the maximum height is 136 ft