Question

A pitcher claims he can throw a 0.145-kg baseball with as much momentum as a 3.00-g bullet moving with a speed of 1.50 3 103 m/s. (a) What must the baseball’s speed be if the pitcher’s claim is valid? (b) Which has greater kinetic energy, the ball or the bullet?

Answer 1

(a) Speed of baseball is 31.03 m/s

(b) Bullet has greater kinetic energy than baseball

Step-by-step explanation:

Given :

Mass of baseball, M = 0.145 kg

Mass of bullet, m = 3 g = 3 x 10⁻³ kg

Speed of bullet, v = 1.50 x 10³ m/s

Let u m/s be the speed of the baseball.

(a)

According to the problem, momentum of bullet and momentum of baseball is same. So,

M x u = m x v

`u=(m* v)/(M)`

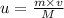
Substitute the values of m, M and v in the above equation.

`u=(3*10^(-3)*1.50*10^(3) )/(0.145)`

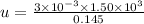
u = 31.03 m/s

(b) Kinetic energy of baseball is given by the relation :

`K_(1)=(1)/(2) Mv^(2)`

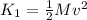
Substitute the values of M and v in the above equation.
`K_(1)=(1)/(2) * 0.145*(31.03)^(2)`

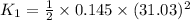
K₁ = 69.80 J

Kinetic energy of bullet is given by the relation :

`K_(2)=(1)/(2) m u^(2)`

Substitute the values of M and v in the above equation.

`K_(2)=(1)/(2)*3*10^(-3)*(1.50*10^(3) )^(2)`

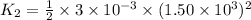
K₂ = 3375 J

Since, K₂ is greater than K₁, hence bullet has greater kinetic energy than baseball.