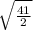Answer:
x = - 4 ±
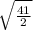
Explanation:
Given
f(x) = 2x² + 16x - 9
To find the zeros, let f(x) = 0, that is
2x² + 16x - 9 = 0
Using the method of completing the square
The coefficient of the x² term must be 1
Factor out 2 from 2x² + 16x
2(x² + 8x) - 9 = 0
add/ subtract ( half the coefficient of the x- term )² to x² + 8x
2(x² + 2(4)x + 16 - 16) - 9 = 0
2(x + 4)² - 32 - 9 = 0
2(x + 4)² - 41 = 0 ( add 41 to both sides )
2(x + 4)² = 41 ( divide both sides by 2 )
(x + 4)² =
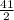 ( take the square root of both sides )
( take the square root of both sides )
x + 4 = ±
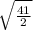 ( subtract 4 from both sides )
( subtract 4 from both sides )
x = - 4 ±
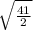
Thus
x = - 4 +
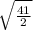 or x = - 4 +
or x = - 4 +