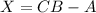Looks like the matrix equation is supposed to be
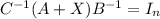
where
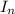 presumably denotes the
presumably denotes the
 identity matrix.
identity matrix.
Since
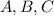 are all invertible, we have by multiplying on the left by
are all invertible, we have by multiplying on the left by
 ,
,
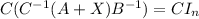
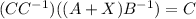

then multiplying on the right by
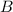 ,
,
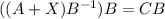
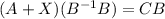

and finally subtracting
 from both sides to end up with
from both sides to end up with