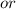Question

During halftime of a soccer ​game, a sling shot launches​ T-shirts at the crowd. A​ T-shirt is launched from a height of 4 feet with an initial upward velocity of 64 feet per second. The​ T-shirt is caught 49 feet above the field. How long will it take the​ T-shirt to reach its maximum​ height? What is the maximum​ height? What is the range of the function that models the height of the​ T-shirt over​ time?

Answer 1

`or`The general equation that represents height of the t-shirt as a function of time:

`64/4=16 \\f(t)= -16t^(2) +64t+5`

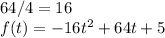
The maximum height of the t-shirt:

`t=(-64)/(2(-16)) =2\\f(2.25)=-16(2)^(2) +64(2)+5\\= 69 feet`

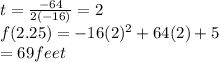
How long will it take the t-shirt to reach its maximum height:

2 seconds

How long does it take for the t-shirt to reach the crowd:

`49=-16t^(2) +69t+5\\o=-16t^(2)+69t-30\\\\\frac{-64+\sqrt[]{-64^(2-4(-16)(-30))} }{2(-16)} = .54`
`seconds` or
`3.04`
`seconds`

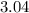
The range of this graph in inequality and interval notation:

`heights(y)\\5\leq y\leq 69 [5,86]`