Answer:
(1) Null Hypothesis,
 :
:
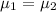
Alternate Hypothesis,
 :
:
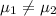
(2) Test statistics = -1.48
(3) At the 0.05 significance level, Fair field conclude that the population means are same.
Explanation:
Let
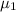 = mean annual family income for 12 people making inquiries at the first development
= mean annual family income for 12 people making inquiries at the first development
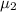 = mean annual family income for 24 people making inquiries at the second development
= mean annual family income for 24 people making inquiries at the second development
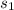 = standard deviation of annual family income for 12 people making inquiries at the first development
= standard deviation of annual family income for 12 people making inquiries at the first development
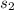 = standard deviation of annual family income for 24 people making inquiries at the second development
= standard deviation of annual family income for 24 people making inquiries at the second development
 = sample of people of first development i.e. 12
= sample of people of first development i.e. 12
 = sample of people of second development i.e. 24
= sample of people of second development i.e. 24
(1) Null Hypothesis,
 :
:
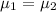 {population means are same}
{population means are same}
Alternate Hypothesis,
 :
:
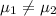 {population means are different}
{population means are different}
DECISION RULE ;
- If the test statistics is less than the critical value of t from table at 5% significance level, then we will accept null hypothesis,
 .
. - If the test statistics is more than the critical value of t from table at 5% significance level, then we will reject null hypothesis,
 .
.
(2) The test statistics is given by;
 ~
~
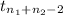
where,
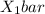 = Sample mean income of people at first development
= Sample mean income of people at first development
= $153,000
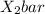 = Sample mean income of people at second development = $171,000
= Sample mean income of people at second development = $171,000
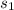 = $42,000 and
= $42,000 and
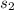 = $30,000
= $30,000
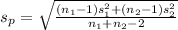 =
=
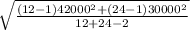 = 34344.30
= 34344.30
Test statistics =
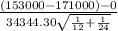 ~
~
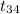
= -1.48
(3) At 5% level of significance, t table gives critical value of 2.032 at 34 degree of freedom.Since our test statistics is less than the critical value of t so considering our decision rule, we will accept null hypothesis.
And conclude that population means are same.