Answer:
(a) $200 today is equivalent to having $208 in one year with 4% interest rate.
(b) $192.31 today would be equivalent to $200 in one year with 4% interest rate.
(c) true
Step-by-step explanation:
(a) since simple interest is given as:
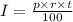
Where p = the principal amount
r = interest rate
t = number of years
Now, from the question our principal is $200 since we are to calculate the interest on it, and our rate is 4% as obvious from the question and as well as our time is 1year. Substituting these to the interest formula we get:
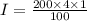
And that gives $8 as the interest, therefore in one year the amount we shall have will be: $200 + $8 = $208
(b) assuming we have 200 as the amount, we have now, we can get the principal we had last year at 4% interest with the following
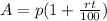
Now our amount is assumed to be $200 for 1year at 4% interest rate, therefore we have:
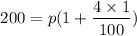
This gives:
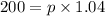
Which gives
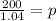
p = $192.31(to the nearest cents)
(c) The statement is obviously true, because the amount we buy things today are more expensive than the amount we bought them in previous years, so having $200 today is preferable because the interest on it is just so small and would be a waste of resources.