Question

A ship sets sail Cape Ann, Massachusetts, heading due north at 7.00 m/s relative to the water. The local ocean current is 1.50 m/s in a direction 40.0o north of east. What is the velocity of the ship relative to the Earth

Answer 1

`\vec{v} = 1.15 \hat{i} + 7.964 \hat{j}`

`|v| = 8.05 m/s`

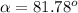`\alpha = 81.78^o` north of east

Step-by-step explanation:

Let i and j be the unit components of east and north directions, respectively. We can calculate the velocity components of ocean current:

`\vec{v_o} = 1.5cos40^0 \hat{i} + 1.5sin40^0\hat{j}`

`\vec{v_o} = 1.15 \hat{i} + 0.964 \hat{j}`

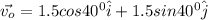
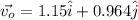
The velocity vector for ship with respect to water is

`\vec{v_s} = 7\hat{j}`

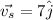
So the velocity vector of the ship with respect to Earth is the sum vector of velocity of ship with respect to water and velocity of water with respect to Earth

`\vec{v} = \vec{v_s} + \vec{v_o} = 7\hat{j} + 1.15 \hat{i} + 0.964 \hat{j} = 1.15 \hat{i} + 7.964 \hat{j}`

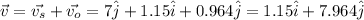
This vector would have a magnitude and direction of:

`|v| = √(v_j^2 + v_i^2) = √(7.964^2 + 1.15^2) = √(63.425296 + 1.3225) = √(64.747796) = 8.05`

`tan\theta = (v_j)/(v_i) = (7.964)/(1.15) = 6.93`

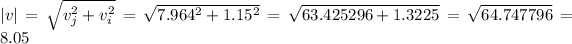
`\alpha = tan^(-1)6.93 = 1.43 rad \approx 81.78^o` north of east