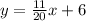Question

Type the correct answer in each box. Use numerals instead of words. If necessary, use / for the fraction bar(s). A construction crew is extending the length of the center line on a highway. The length of the line starts out as 6 meters long, which is represented on a coordinate plane as the point (0,6). The crew works for 20 minutes and the line is now 17 meters long, which is represented as the point (20,17). Complete the equation that represents the relationship between x, the number of minutes spent working, and y, the length of the line, in meters.

Answer 1

`y=(11)/(20)x+6`

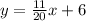
Explanation:

Given:

Two points are given

x = number of minutes

y = length of the lin

`(x_(1),y_(1) )` ⇒ (0, 6)

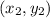`(x_(2),y_(2) )` ⇒ (20, 17)

We need to find the equation that represents the relationship between x, y.

Solution:

Using slope formula to find the slope of the equation of the line.

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

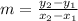
Substitute
`(x_(1),y_(1) )` = (0, 6) and
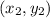`(x_(2),y_(2) )` = (20, 17) in above equation.

`m=(17-6)/(20-0)\\m=(11)/(20)`

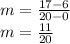
So, slope of the line
`m = (11)/(20)`

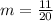
Using point slope formula.

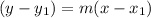`(y-y_(1))=m(x-x_(1))` ------------(1)

Where, m = slope of the line

Substitute
`(x_(1),y_(1) )` ⇒ (0, 6) and
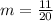`m = (11)/(20)` in equation 1.

`(y-6)=(11)/(20) (x-0)`

`y-6=(11)/(20)x`

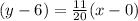
Add 6 both side of the equation.

`y-6+6=(11)/(20)x+6`

`y=(11)/(20)x+6`

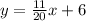
Therefore, the equation that represents the relationship between x and y is written as:

`y=(11)/(20)x+6`