x intercept of CD is (17, 0)
Point (-2, 19) lies on CD
Solution:
CD is perpendicular to AB and passes through point C(5, 12)
Coordinates of A and B are (-10, -3) and (7, 14)
Find slope of AB
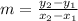
From given,
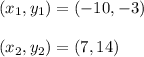
Substituting we get,

CD is perpendicular to AB
We know that,
Product of slope of AB and slope of line CD which is perpendicular to AB is equal to -1
Therefore,
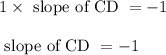
The equation of CD in slope intercept form is:
y = mx + c --------- eqn 1
Where,
m is the slope
c is the y intercept
Substitute m = -1 and (x, y) = (5, 12) in eqn 1
12 = -1(5) + c
c = 12 + 5
c = 17
Substitute m = -1 and c = 17 in eqn 1
y = -x + 17 ------ eqn 2
The x-intercept is found by setting y equal to 0
0 = -x + 17
x = 17
Thus x intercept of CD is (17, 0)
For the second part, we just plug in the different points and see if the equation is true:
Substitute (x, y) = (-5, 24) in eqn 2
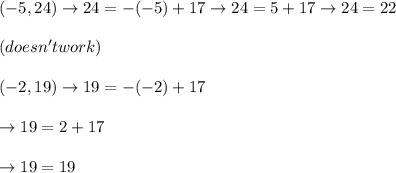
Thus, Point (-2, 19) lies on CD