Express the complex number in trigonometric form. 4i

Question

asked Aug 6, 2021 32.9k views

1 Answer

Vanitas · Answer 1 · 2021-08-11T05:27:47+0000

Question: Express
in trigonometric form.

Answer:

A few ways to express this is:

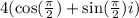
$4(\cos((\pi)/(2))+\sin((\pi)/(2))i)$

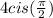
$4cis((\pi)/(2))$

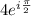
$4e^{i(\pi)/(2)}$

Choose the notation your class is using.

Explanation:

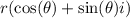$r(\cos(\theta)+\sin(\theta)i)$ is trigonometric form.

Distributing
to terms inside the ( ) gives:

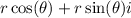$r\cos(\theta)+r\sin(\theta)i$ .

Our number has no real part so we are just trying to find
and
$\theta$ such that
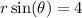$r\sin(\theta)=4$ .

Since we want
$\cos(\theta)=0$ , we will have to
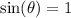$\sin(\theta)=1$ (or -1).

This happens at
$(\pi)/(2)$ .

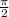This means
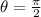$\theta=(\pi)/(2)$ .

So while
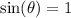$\sin(\theta)=1$ this forces
r=4

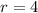
The trigonometric form is
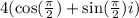$4(\cos((\pi)/(2))+\sin((\pi)/(2))i)$ .

Some people like to express this as
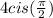$4cis((\pi)/(2))$ which is way shorter.

Some people might also express this as
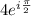$4e^{i(\pi)/(2)}$ .