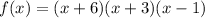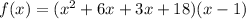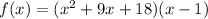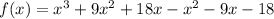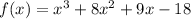Answer:
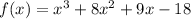
Explanation:
If
 is a zero of
is a zero of
 , then
, then
 is a factor of
is a factor of
 .
.
Since -6,-3, and 1 are zeros, then we have the following factors of
 :
:
 ,
,
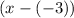 , and
, and
 .
.
Let's put it together.
 , where
, where
 is the leading coefficient and we are given to make it equal to 1, has zeros -6,-3, and 1.
is the leading coefficient and we are given to make it equal to 1, has zeros -6,-3, and 1.
We now need to put the factored form of
 into standard form by using multiplication and combining of like terms.
into standard form by using multiplication and combining of like terms.