Answer:
The answer is c.) 0.6 and 0.14
Explanation:
Given population has a size of 320.
The proportion of population = 0.6
The mean of population, p = 0.6
The mean of sample,
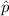 = 0.6
= 0.6
Therefore
 = 1 -
= 1 -
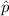 = 1 - 0.6 = 0.4
= 1 - 0.6 = 0.4
The sample size = 12
Therefore the standard deviation of the sample,
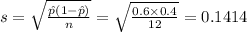
The mean and the standard deviation for a sample size of 12 are 0.6 and 0.14 respectively.
The answer is c.) 0.6 and 0.14