Question

A formula for the relationship between weight and blood pressure in children is given by the formula below where P(x) is measured in millimeters of mercury and x is measured in pounds. Use the formula to answer the questions. P(x) = 12.9(9 + ln x) 10 x 100 What is the rate of change of blood pressure with respect to weight at the 50-pound weight level? The rate of change at the 50-pound weight level is approximately .26 mm/pound. (Do not round until the final answer. Then round to the nearest hundredth as needed.) What is the rate of change of blood pressure with respect to weight at the 80-pound weight level? The rate of change at the 80-pound weight level is approximately mm/pound. (Do not round until the final answer. Then round to the nearest hundredth as needed.)

Answer 1

(a) The rate of change of blood pressure with respect to 50 pounds weight level is 0.26mm/pound. (To the nearest hundredth)

(b) The rate of change of blood pressure with respect to 80 pounds weight level is 0.16mm/pound. (To the nearest hundredth)

Explanation:

The formula relating weight and blood pressure is given by:

P(x) = 12.9(9 + lnx) for
`10\leq x\leq 100`

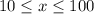
The rate of change of one thing with respect to the other is the derivative of the first with respect to the second. Therefore, for the purpose of this question, the rate of change of blood pressure P(x) with respect to the weight (x) is the derivative of P(x) with x.

Rate of change =
`(dy)/(dx) = (dP(x))/(dx)`

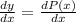
Differentiating the equation given with respect to x i.e

P(x) = 12.9(9 + lnx)

expanding the bracket by multiplying the characters in the bracket with the character outside the bracket, we have:

P(x) = 12.9 x 9 + 12.9 x lnx

P(x) = 116.1 + 12.9lnx,

differentiating this P(x), Recall from standard derivative,

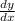`(dy)/(dx)` of a constant is zero

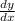`(dy)/(dx)` of lnx is
`(1)/(x)`

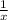
Applying this to the P(x) with x

`(d(P(x)))/(dx) = (d(116.1))/(dx) + (d(12.9lnx))/(dx)`

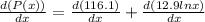
= 0 +
`(12.9)/(x)`

`(dP(x))/(dx) = (12.9)/(x)`

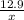
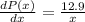
(a) When weight x = 50 pounds,

`(d(P(x)))/(dx) = (12.9)/(50)` = 0.258

= 0.26mm/pound (To the nearest hundredth)

(b) When weight x = 80 pounds,

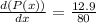`(d(P(x)))/(dx) = (12.9)/(80)` = 0.16125

= 0.16mm/pound (To the nearest hundredth)

From our result, it is shown that the rate of change of blood pressure with respect to weight reduces with increase in weight.

Answer 2

The rate of change of blood pressure at the 50-pound weight level is 0.26

The rate of change of blood pressure at the 80-pound weight level is 0.16

Explanation:

We have, P(x) = 12.9(9 + ln x)

We need to compute the rate of change of blood pressure P(x) so, we will differentiate the function P(x) with respect to x.

P(x) = 12.9(9 + ln x)

P(x) = 116.1 + 12.9ln x

Differentiating with repect to x:

`(d(P(x)))/(dx)` = 0 + 12.9 (
`(1)/(x)`)

`(d(P(x)))/(dx)` = 12.9 (
`(1)/(x)`)

The differential of ln x is
`(1)/(x)` and the differential of constant terms is 0.

The rate of change of blood pressure at the 50-pound weight level can be calculate by substituting 50 in place of x, so

`(d(P(x)))/(dx)` = 12.9 (
`(1)/(x)`)

= 12.9 * (1/50)

= 0.258

`(d(P(x)))/(dx)` = 0.26

Similarly, The rate of change of blood pressure at the 80-pound weight level can be calculate by substituting 80 in place of x, so

`(d(P(x)))/(dx)` = 12.9 (
`(1)/(x)`)

= 12.9 * (1/80)

= 0.16125

`(d(P(x)))/(dx)` = 0.16