Answer:
F = 752.68 N (force required to stop)
Step-by-step explanation:
Let's first find the total speed the cat was travelling at when it hit the ground.
This can be calculated by the following motion equation:
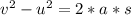
Here u = initial velocity = 0
a = acceleration due to gravity = 9.81 m/s
s = Distance covered from branch to ground = 2 m
Solving for v , we get 6.2641 m/s.
Let's solve the same motion equation again to find the deceleration required:
v^2 - u^2 = 2*a*s
where u = initial velocity = 6.2641 m/s
v = final velocity = 0 m/s
s = 0.11 m or 11 cm
Solving for a, we get - 178.36 m/s^2
Finally we can plug this acceleration into Newton's force equation : F = m*a
The force needs to incorporate the weight of the cat as well, so we can divide this into:
F1 = weight of cat = 4 * 9.81 = 39.24
F2 = average force required to stop
Newton's equation now becomes:
F1 - F2 = mass * acceleration
39.24 - F2 = 4 * (-178.36)
F2 = 752.68 N (force required to stop)