Answer:
The quantity of P(t) in the lake is
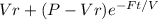 litres where V is the amount of water in litres, r is the concentration of the flow in, P is the initial amount , F is the rate of flow in/out and t is time.
litres where V is the amount of water in litres, r is the concentration of the flow in, P is the initial amount , F is the rate of flow in/out and t is time.
Explanation:
The equation is given
Rate of Change of Amount=Flow Rate(in)*(Conc. of flow in)-Flow Rate(out)*(Conc. at any given time)
Here
Change of Amount is given P'(t)
Flow Rate(in) and Flow Rate(out) are given F
The conc. at any time is given P(t)/V
The conc. of the Flow in is given r
The initial concentration is given P
so the equation becomes
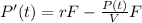
Now this equation in the standard form of the linear differential equation is as below and the integrating factor is
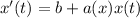
Here
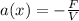
So integrating factor is
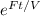
Now the equation is
![P(t)=e^(-Ft/V) * \int{e^(F/V)Frdt} \\P(t)=e^(-Ft/V) *[Fr(V)/(F)e^(Ft/V)+C]\\P(t)=Vr+Ce^(-Ft/V)](https://img.qammunity.org/2021/formulas/mathematics/college/8xum1ral5d1burfmqa4bxnwq68c2e01nli.png)
As the initial condition is
As P(0)=P so by substituting this in the equation gives
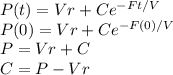
Putting this in the above differential equation
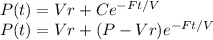
So the amount P(t) in the lake is
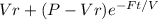 litres where V is the amount of water in litres, r is the concentration of the flow in, P is the initial amount, F is the rate of flow in/ out and t is time.
litres where V is the amount of water in litres, r is the concentration of the flow in, P is the initial amount, F is the rate of flow in/ out and t is time.