Question

A certain sound source is increased in sound level by 21 dB. By what multiple is (a) its intensity increased and (b) its pressure amplitude increased?

Answer 1

(a). The Final intensity is 126 times of initial intensity.

(b). The pressure amplitude increased is 11.22 times of initial pressure.

Step-by-step explanation:

Given that,

Sound level = 21 dB

(a). We need to calculate the increases increases

Using sound level equation

`\beta=10dB log((I)/(I_(0)))`

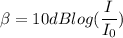
The intensity level for original sound level is

`\beta_(1)= 10dB log((I_(1))/(I_(0)))`

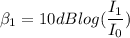
The intensity level for final sound level is

`\beta_(2)= 10dB log((I_(2))/(I_(0)))`

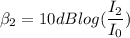
Subtract equation (I) from equation (2)...

`\beta_(2)-\beta_(1)=10dB log((I_(2))/(I_(0)))-10dB log((I_(1))/(I_(0)))`

`\beta_(2)-\beta_(1)=10dB log(((I_(2))/(I_(0)))/((I_(1))/(I_(0))))`

`\beta_(2)-\beta_(1)=10dB log((I_(2))/(I_(1)))`

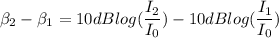
Put the value into the formula

`21 dB=10 dB log((I_(2))/(I_(1)))`

`(21)/(10)=log((I_(2))/(I_(1)))`

`I_(2)=10^(2.1)I_(1)`

`I_(2)=126 I_(1)`

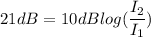
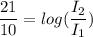
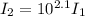
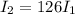
The Final intensity is 126 times of initial intensity.

(b). We need to calculate the pressure amplitude increased

Using formula of pressure

`P_(2)=\sqrt{I_(2)}`

`P_(2)=\sqrt{126I_(1)}`

`P_(2)=11.22P_(1)`

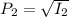
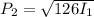
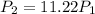
The pressure amplitude increased is 11.22 times of initial pressure.

Hence, (a). The Final intensity is 126 times of initial intensity.

(b). The final pressure amplitude is 11.22 times of initial pressure.