Question

When the shaded region below is rotated about the x-axis the resulting solid is a circular cone with radius 2 m and height 3 m. Use the disk or shell method to find the volume of this cone

Answer 1

Therefore, the volume of the cone is V=4π.

Explanation:

From task we have a circular cone with radius 2 m and height 3 m. We use the disk method to find the volume of this cone.

We have the formula:

`\boxed{V=\int_0^h\pi \cdot \left((r)/(h)x\right)^2\, dx}`

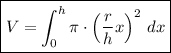
We know that r=2 and h=3, and we get:

`V=\int_0^3\pi \cdot \left((2)/(3)x\right)^2\, dx\\\\V=\int_0^3 \pi (4)/(9)x^2\, dx\\\\V= (4\pi)/(9) \int_0^3 x^2\, dx\\\\V= (4\pi)/(9) \left[(x^3)/(3)\right]_0^3\, dx\\\\V= (4\pi)/(9)\cdot 9\\\\V=4\pi`

Therefore, the volume of the cone is V=4π.