Question

Two automobiles A and B, of mass mA and mB, respectively are traveling in opposite directions when they collide head on. The impact is assumed to be perfectly plastic, and it is assumed that the energy absorbed by each vehicle is equal to its loss of kinetic energy with respect to the moving frame of reference attached to the mass center of the two-vehicle system. Denoting EA and EB, respectively, the energy absorbed by automobile A and by automobile B.

a. Show that that is, the amount of energy absorbed by each vehicle is inversely proportional to its mass.
b. Compute EA and EB, knowing that ma = 1600 kg and mg = 900 kg and that the speeds of A and B are, respectively, 90 km/h and 60 km/h.

Answer 1

Part a: The energy absorbed is inversely proportional to its mass.

Part b: The energy absorbed by auto A is 180kJ and that of energy absorbed by the auto B is 320 kJ.

Step-by-step explanation:

Part a:

Consider the case before collision which is given as

Let v be the velocity of center of Mass G

By the conservation of momentum

`m_Av_A+m_B(-v_B)=(m_A+m_B)v\\v=(m_Av_A-m_Bv_B)/(m_A+m_B)`

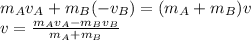
Now the relative motion of A wrt G, the velocity is given as

`v_(A/G)=v_A-v\\v_(A/G)=v_A-(m_Av_A-m_Bv_B)/(m_A+m_B)\\v_(A/G)=((m_Av_A-m_Bv_A)-(m_Av_A-m_Bv_B))/(m_A+m_B)\\v_(A/G)=((m_Av_A-m_Bv_A-m_Av_A+m_Bv_B)/(m_A+m_B)\\v_(A/G)=(m_Bv_B+m_Bv_A)/(m_A+m_B)\\`

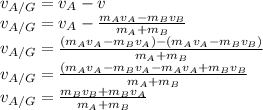
Similarly the relative motion of auto B wrt G, the velocity is given as

`v_(B/G)=-(m_Av_B+m_Av_A)/(m_A+m_B)\\`

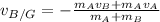
Now the Kinetic Energies are given as

For the auto A

`K.E_(A/G)=(1)/(2)m_Av_(A/G)^2\\K.E_(A/G)=(1)/(2)[m_A(m_Bv_B+m_Bv_A)/(m_A+m_B)]^2\\K.E_(A/G)=(1)/(2)(m_Am_B^2(v_A+v_B)^2)/((m_A+m_B)^2)`

For the auto B

`K.E_(B/G)=(1)/(2)m_Bv_(B/G)^2\\K.E_(B/G)=(1)/(2)[m_B(m_Av_B+m_Av_A)/(m_A+m_B)]^2\\K.E_(B/G)=(1)/(2)(m_Bm_A^2(v_A+v_B)^2)/((m_A+m_B)^2)`

Now the case after collision relates that

As there is no external force the G is moving along the whole system thus

`v_A'=v_B'=v`

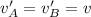
Or

`v_(A/G)' =v_(B/G)' =v-v=0`

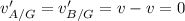
Similarly

`K.E_(A'/G)=(1)/(2)v'_(A/G)^2=0\\K.E_(B'/G)=(1)/(2)v'_(B/G)^2=0`

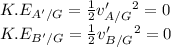
So the energy absorbed are given as

`E_A=K.E_(A/G)=(1)/(2)(m_Am_B^2(v_A+v_B)^2)/((m_A+m_B)^2)\\E_B=K.E_(B/G)=(1)/(2)(m_Bm_A^2(v_A+v_B)^2)/((m_A+m_B)^2)`

Now taking the ratio of these values as

`(E_A)/(E_B)=((1)/(2)(m_Am_B^2(v_A+v_B)^2)/((m_A+m_B)^2))/((1)/(2)(m_Bm_A^2(v_A+v_B)^2)/((m_A+m_B)^2))\\(E_A)/(E_B)=(m_Am_B^2)/(m_Bm_A^2)\\(E_A)/(E_B)=(m_B)/(m_A)`

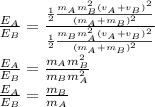
So The energy absorbed is inversely proportional to its mass.

Part b

Mass of car A=m_a=1600 kg

Mass of car B=m_b=900 kg

Velocity of car A=v_a=90 km/h=25 m/s

Velocity of car B=v_b=60 km/h=16.67 m/s

Now the energy absorbed by the car A is given as

`E_A=K.E_(A/G)=(1)/(2)(m_Am_B^2(v_A+v_B)^2)/((m_A+m_B)^2)\\E_A=(1)/(2)(1600* 900^2(25+16.67)^2)/((1600+900)^2)\\E_A=(1)/(2)(1600* 900^2(25+16.67)^2)/((1600+900)^2)\\E_A=180 kJ`

Now the energy absorbed by the car B is

`E_B=K.E_(B/G)=(1)/(2)(m_Bm_A^2(v_A+v_B)^2)/((m_A+m_B)^2)\\E_B=(1)/(2)(1600^2* 900(25+16.67)^2)/((1600+900)^2)\\E_B=(1)/(2)(1600^2* 900(25+16.67)^2)/((1600+900)^2)\\E_B=320 kJ`

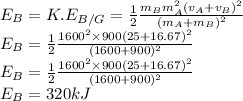
So the energy absorbed by auto A is 180kJ and that of energy absorbed by the auto B is 320 kJ.

Answer 2

EA = 180KJ

EB = 320KJ

Where EA and EB, are the energy absorbed by automobile A and by automobile B

Step-by-step explanation:

The concept of momentum and energy is applied here as it relates to elastic and inelastic collision.

The detailed steps, mathematical manipulation and appropriate substitution is as shown in the attached file.