Answer:
d. The interval contains only negative numbers. We cannot say at the required confidence level that one region is more interesting than the other.
Explanation:
Hello!
You have the data of the chemical measurements in two independent regions. The chemical concentration in both regions has a Gaussian distribution.
Be X₁: Chemical measurement in region 1 (ppm)
Sample 1
n= 12
981 726 686 496 657 627 815 504 950 605 570 520
μ₁= 678
σ₁= 164
Sample mean X[bar]₁= 678.08
X₂: Chemical measurement in region 2 (ppm)
Sample 2
n₂= 16
1024 830 526 502 539 373 888 685 868 1093 1132 792 1081 722 1092 844
μ₂= 812
σ₂= 239
Sample mean X[bar]₂= 811.94
Using the information of both samples you have to determina a 90% CI for μ₁ - μ₂.
Since both populations are normal and the population variances are known, you can use a pooled standard normal to estimate the difference between the two population means.
[(X[bar]₁-X[bar]₂)±
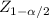 *
*
 ]
]
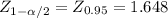
[(678.08-811.94)±1.648*
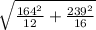 ]
]
[-259.49;-8.23]ppm
Both bonds of the interval are negative, this means that with a 90% confidence level the difference between the population means of the chemical measurements of region 1 and region 2 may be included in the calculated interval.
You cannot be sure without doing a hypothesis test but it may seem that the chemical measurements in region 1 are lower than the chemical measurements in region 2.
I hope it helps!