Question

The Stomping Elephants volleyball team plays 30 matches in a week-long tournament. On average, they win 4 out of every 6 matches. What is the mean for the number of matches that they win in the tournament?

Answer 1

The mean for the number of matches that they win in the tournament is 20.

Explanation:

For each match, there are only two possible outcomes. Either they win, or they do not. The probability of winning a match is independent from other matches. So we use the binomial probability distribution to solve this problem.

Binomial probability distribution

Probability of exactly x sucesses on n repeated trials, with p probability.

The expected value of the binomial distribution is:

`E(X) = np`

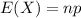
The Stomping Elephants volleyball team plays 30 matches in a week-long tournament.

This means that
`n = 30`

On average, they win 4 out of every 6 matches.

This means that
`p = (4)/(6) = (2)/(3)`

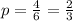
What is the mean for the number of matches that they win in the tournament?

`E(X) = np = 30(2)/(3) = 20`

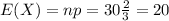
The mean for the number of matches that they win in the tournament is 20.