Question

Joe is building a rectangle a pool in his yard. He knows that the perimeter of the pool must be 88 feet, but he wants the surface of the pool to have the largest possible area. What length and width should you choose for the pool

Answer 1

For maximum surface area the width of the pool is 22 feet and length of the pool be 22 feet.

Explanation:

Here the Perimeter of the rectangular pool is 88 feet.

Let us suppose that the length of the pool be a feet and width of the pool be b feet.

Therefore the perimeter of the pool is = 2(a+b)

Therefore, according to the condition,

2(a+b) = 88

or, a+b = 44

or, a= 44 - b

Now the area of the pool is = ab = (44-b)b (since, a= 44 - b)

Let us suppose y = (44-b)b

or,y =
`44b - b^(2)`.

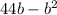We have to find the maximum value of the surface area y.

Now, differentiating y with respect b, we get

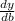`(dy)/(db)` = 44 - 2b

Again, differentiating
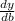`(dy)/(db)` with respect b, we get

`(d^(2)y )/(db^(2) )` = -2 , which is always less than zero for any value of b.

therefore, y has maximum value at 44 - 2b = 0

or, b= 22.

Therefore for maximum surface area the width of the pool is 22 feet and length of the pool be (44-22) =22 feet.