Question

What is the measure of angle x? Show work.

Answer 1

∠x = 67°

Explanation:

From the Law of Sines,

we know that :

• sin(A) / a = sin(B) / a

Here we have :

• a = 12
• b = 18
• ∠A = 38°
• ∠B = x°

On substituting,

• sin38° / 12 = sinx° / 18
• sinx° = 3/2 x sin38°
• x = 3/2sin38° x sin⁻¹
• ∠x = 67°

Answer 2

x = 67° (nearest whole degree)

Explanation:

Sine Rule

`\sf (sin(A))/(a)= (sin(B))/(b)= (sin(C))/(c)`

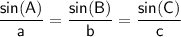
where A, B and C are the angles, and a, b and c are the sides opposite the angles

Given information

From inspection of the triangle:

• A = 38°
• a = 12
• B = x°
• b = 18

Finding x:

Substitute given values into the formula and solve for x:

`\sf \implies (sin(38))/(12)= (sin(x))/(18)`

`\sf \implies 18\cdot(sin(38))/(12)= sin(x)`

`\sf \implies sin(x)=\frac32sin(38)`

`\sf \implies x=sin^(-1)\left(\frac32sin(38)\right)`

`\sf \implies x=67.44208077...`

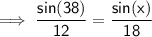
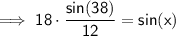
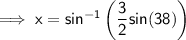
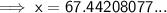
Final Solution

x = 67° (nearest whole degree)