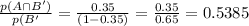Question

The probability that event A happens is 0.45. The probability that event B happens is 0.35. The probability that at least one of these two events happens is 0.70. What is the probability: (a) that A happens but B does not? (b) exactly one of the two events happens? (c) that event A happens given that event B does not happen?

Answer 1

a.) The probability that A happens but B does not is 0.35

b.) The probability exactly one of these two events happens is 0.6

c.) The probability that event A happens given that event B does not happen is 0.5385.

Explanation:

The probability that event A happens , p(A) = 0.45

The probability that event B happens, p(B) = 0.35

The probability that at least one of these two events happens, P(A∪B) = 0.7

a.) The probability that A happens but B does not is

= p(A ∩ B')

= p(A) - p(A ∩ B)

= 0.45 - {p(A) + p(B) - p(A∪B)}

= 0.45 - {0.45 + 0.35 - 0.7}

= 0.45 - 0.1

= 0.35

b.) the probability exactly one of these two events happens

= p(A∩B') + p(A' ∩ B)

= P(A) + p(B) - 2p(A∪B)

= 0.45 + 0.35 - (2×0.1)

= 0.6

c.) the probability that event A happens given that event B does not happen

= p( A | B' )

=
`(p(A \cap B'))/(p(B') = (0.35)/((1 - 0.35)) = (0.35)/(0.65) = 0.5385`