Question

A cylinder and a cone have the same volume. The cylinder has a diameter of 4 inches and a height of 3 inches. The cone has a diameter of 6 inches. What is the height of the cone?

Answer 1

• The height of the cone is 4 inches.

Solution :

We are given a cylinder and a cone of same volume i.e

`\longrightarrow` Volume of cone = Volume of cylinder

And also the diameter of the cylinder is given 4 inches

`\longrightarrow` Radius =
`\sf (Diameter)/(2)`

`\longrightarrow` Radius =
`\sf(4)/(2)`

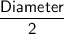
`\longrightarrow` Radius = 2 inches

The height of cylinder is 3 inches. And the diameter of the cone is 6 inches

`\longrightarrow` Radius =
`\sf(Diameter)/(2)`

`\longrightarrow` Radius =
`\sf(6)/(2)`

`\longrightarrow` Radius = 3 inches

First, let us recall the Formulas of volume of cylinder and cone:

`\quad\longrightarrow\quad \sf {Cone = (1)/(3)\pi r^2 h }`

`\quad\longrightarrow\quad \sf {Cylinder = \pi r^2 h }`

Now, we know that the volume of cone is equal to the volume of cylinder

`\implies\quad \sf{(1)/(3)\pi r^2 h = \pi r^2 h }`

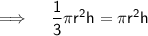
On putting the values:

`:\implies\quad \sf{(1)/(3)\pi * 3^2 * h = \pi * 2^2 * 3 }`

`:\implies\quad \sf{\frac{1}{\cancel{3}}* 3.14 *\cancel{ 9 }* h = 3.14 * 4 * 3 }`

`:\implies\quad \sf{3.14 * 3 * h = 3.14 * 12 }`

`: \implies\quad \sf{ h = \frac{ \cancel{3.14} * 12 }{ \cancel{3.14} * 3} }`

`:\implies\quad \sf{ h =\cancel{ (12)/(3)}}`

`:\implies\quad \underline{\underline{\pmb{\sf{h = 4 inches }}} }`

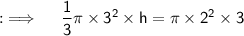
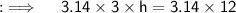
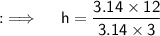
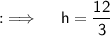
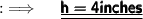
‎ㅤ‎ㅤ‎ㅤ‎ㅤ‎ㅤ~Hence the height of the cone is 4 inches.