Question:
Which equation can be solved by using this system of equations?
y = 3x^3 - 7x^2 + 5
y = 7x^4 + 2x
A.) 3x^3 - 7x^2 +5 = 0
B.) 3x^3 - 7x^2 + 5 = 7x^4 +2x
C.) 7x^4 +2x = 0
D.) 7x^4 +3x^3 -7x^2 +2x + 5 = 0
Answer:
Option B:
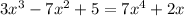 is used to solve this system of equations.
is used to solve this system of equations.
Step-by-step explanation:
The two equations are
 and
and

Using substitution method, let us substitute
 in the equation
in the equation
 , we get,
, we get,
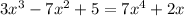
Thus,
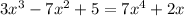 is used to solve this system of equations.
is used to solve this system of equations.
Hence, Option B is the correct answer.