Answer:
Step-by-step explanation:
Part (a):
Using the equation of pressure gradient for velocity, along the streamline:

where,
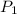 = Required pressure
= Required pressure
 = Atmospheric pressure
= Atmospheric pressure
 = Velocity of the Flow
= Velocity of the Flow
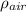 = Density of air at 1500m above sea level
= Density of air at 1500m above sea level
Assuming the flow as incompressible, above equation becomes

Substituting values for pressure and density, we get
![P_1 \;= \;14.7(lb)/(in^2) \;+\; (1)/(2)[(0.002376(sl)/(ft^3)).\;(60\;mph)^2]\\\\(Standard\; values\; at\; atmospheric\; conditions)\\\\P_1\;=\;14.7(lb)/(in^2).(144\;in^2)/(ft^2) \;+\; (1)/(2)[(0.002376(sl)/(ft^3)).\;(60\;mph\;.\;(1.4667\; (ft)/(s))/(mph))^2]\\\\P_1\;=\; 2126\;\; lb/ft^2](https://img.qammunity.org/2021/formulas/english/college/uhk5va4wnyrwrabzy6dxaoaocvkv0ajaqt.png)
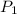 = 14.76 psi
= 14.76 psi
Part (b):
Just replace the value of velocity with 225 mph in part (a). All other values and equations will remain the same.
![P_2 \;= \;14.7(lb)/(in^2) \;+\; (1)/(2)[(0.002376(sl)/(ft^3)).\;(225\;mph)^2]\\\\(Standard\; values\; at\; atmospheric\; conditions)\\\\P_2\;=\;14.7(lb)/(in^2).(144\;in^2)/(ft^2) \;+\; (1)/(2)[(0.002376(sl)/(ft^3)).\;(225\;mph\;.\;(1.4667\; (ft)/(s))/(mph))^2]\\\\P_2\;=\; 2246.17\;\; lb/ft^2](https://img.qammunity.org/2021/formulas/english/college/pdouvjv61751h9ewhosnmla1vr3edij8p1.png)
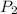 = 15.59 psi
= 15.59 psi
Part (c):
It is given that the speed of sound is 761 mph, which is equal to 1 mach. Therefore, to convert the maximum car speed of 240 mph, we simply divide it by the speed of sound.
Mach Speed =
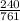
Mach Speed = 0.315 Mach
Part (d):
The difference between compressible and incompressible flow is that, in compressible flow, the density of the fluid varies with pressure, while in incompressible flow, the density remains constant. Compressible flows are usually high speed flows with Mach numbers greater than 0.3 (for example air flow over an aircraft's wing). Therefore, a fluid moving around or below Mach 0.3 is typically considered incompressible, even if it is a gas.
As for our scenario, the mach number for maximum possible speed was only around Mach 0.3, we can assume this as an incompressible flow