Question

A vector has an x component of -24.0 units and a y component of 40.4 units. Find the magnitude and direction of this vector.

Answer 1

The magnitude is 47.0 and the direction is 120.7° above the x-axis counterclockwise.

Step-by-step explanation:

Hi there!

The magnitude of any vector is calculated as follows:

`|v| = \sqrt{x^(2) + y^(2) }`

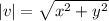
Where:

v = vector.

x = x-component of v.

y = y-component of v.

Then, the magnitude of this vector will be:

`|v| = \sqrt{24.0^(2) + 40.4^(2) } = 47.0`

The magnitude is 47.0

The direction can be found by trigonometry (see attached figure).

According to the trigonometry rules of right triangles (as the one in the figure):

cos θ = adjacent side to the angle θ / hypotenuse

In this case:

adjacent side = x-component of v.

hypotenuse = magnitude of v.

Then:

cos θ = 24.0 / 47.0

θ = 59.3°

Measured in counterclockwise:

180° - 59.3° = 120.7°

The magnitude is 47.0 and the direction is 120.7° above the x-axis counterclockwise.