Answer:
Option B) 3.04
Explanation:
We are given the following in the question:
Number of people interviewed,n = 163
Number of people hire, x = 121
Proportion of people hire =
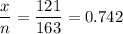
Mean, μ = 3.3
Standard Deviation, σ = 0.4
We are given that the distribution of GPA for the successful interviewees is a bell shaped distribution that is a normal distribution.
Formula:

We have to find the value of x such that the probability is 0.742
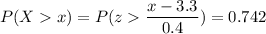


Calculation the value from standard normal z table, we have,
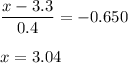
Thus, cut-off GPA should be 3.04.
Option B) 3.04