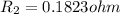Answer:
So after stretching new resistance will be 0.1823 ohm
Step-by-step explanation:
We have given initially length of the wire
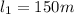
Radius of the wire
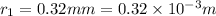
Resistance of the wire initially
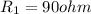
We know that resistance is equal to
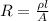 ,here
,here
 is resistivity, l is length and A is area
is resistivity, l is length and A is area
From the relation we can say that
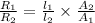
Now length of wire become 6.75 m
Volume will be constant
So
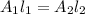
So

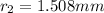
So