Question

Refrigerant 134a (R134a) enters the coils of the evaporator of a refrigeration system as a saturated liquid-vapour mixture at a pressure of 160 kPa. The refrigerant absorbs 180 kJ of heat from the cooled space, which is maintained at -5oC, and leaves as saturated vapour at the same pressure. (a) What is the temperature of the R134a liquid-vapour mixture as it enters the system? (b) What is the specific enthalpy of the R134a liquid-vapour mixture as it exits the system? (c) What is the entropy change of the R134a? (d) What is the total entropy change for this process?

Answer 1

Part a: The temperature of the mixture at 160kPA is 257.68K.

Part b:The specific enthalpy of the mixture as it exits the system is 237.97 kJ/kg.

Part c:The entropy change for the mixture is 0.6999kJ/K

Part d: The total entropy change is 0.027 kJ/K

Step-by-step explanation:

Part a

The temperature is as viewed from the the table of Saturated properties for the Refrigerant 134a for Pressure of 160kPa.

`T_(sat\rightarrow 160kPa)=-15.62^o C\\T_(sat\rightarrow 160kPa)=-15.62+273K\\T_(sat\rightarrow 160kPa)=-15.62^o C\,\, or \,\, 257.28 K`

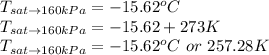
The temperature of the mixture at 160kPA is 257.68K

Part b

For specific enthalpy from the same table of Saturated properties for the Refrigerant 134a for Pressure of 160kPa for the vapour phase which is given as

`h_g=237.97 kJ/kg`

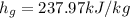
The specific enthalpy of the mixture as it exits the system is 237.97 kJ/kg.

Part c

The entropy change for the mixture is given as

`s=(\Delta Q)/(T)`

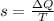
Here

ΔQ is the heat in which is given as 180kJ

T is the temperature of the mixture which is calculated in part a as 257.28K

so the equation becomes.

`s_1=(Q_(in))/(T)\\s_1=(180 kJ)/(257.28K)\\s_1=0.6999 kJ/K`

The entropy change for the mixture is 0.6999kJ/K

Part d

For overall entropy change, the entropy change in the cooled space is also to be calculated such that

Q is the heat in which is given as 180kJ

T is the temperature of the cooled space which is given as -5°C or 268K

so the equation becomes.

`s_2=-( Qout)/(T)\\s_2=-(180 kJ)/(268K)\\s_2=-0.672 kJ/K`

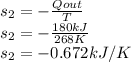
So the total entropy change is

`\Delta s=\Delta s_1+\Delta s_2\\\Delta s_(gen) =0.699-0.672\\\Delta s_(gen) =0.027 kJ/K`

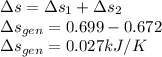
The total entropy change is 0.027 kJ/K