Answer:
Probability = 0.1038 or 10.38%
Explanation:
Given,
Number of sheets n = 250
Mean
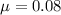
Standard deviation
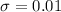
 = Sum of sample items.
= Sum of sample items.
From the Central Limit Theorem we get,
 ~
~
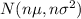
n\mu = 250 × 0.08
= 20

= 250(0.01)²
= 0.025
Therefore,
 ~N(20,0.025)
~N(20,0.025)
The Z-value corresponding to 20.2 :
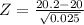
= 1.26
Finally,
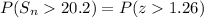
= 1 - 0.8962
= 0.1038
Probability = 0.1038 or 10.38%