Question

A

14.7

g
ice cube is placed into
324

g
of water. Calculate the temperature change in the water upon complete melting of the ice. Hint: Determine how much heat is absorbed by the melting ice and then use
q
=
m
C
Δ
T
to calculate the temperature change. Use the heat of fusion for water to calculate "q"

Answer 1

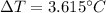`\Delta T=3.615^(\circ)C` is the drop in the water temperature.

Step-by-step explanation:

Given:

• mass of ice,
  `m_i=14.7\ g=0.0147\ kg`

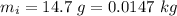
• mass of water,
  `m_w=324\ g=0.324\ kg`

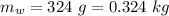
Assuming the initial temperature of the ice to be 0° C.

Apply the conservation of energy:

• Heat absorbed by the ice for melting is equal to the heat lost from water to melt ice.

Now from the heat equation:

`Q_i=Q_w`

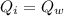
`m_i.L=m_w.c_w.\Delta T` ......................(1)

where:

`L=` latent heat of fusion of ice
`=333.55\ J.g^(-1)`

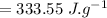
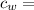`c_w=` specific heat of water
`=4.186\ J.g^(-1).^(\circ)C^(-1)`

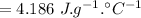
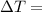`\Delta T=` change in temperature

Putting values in eq. (1):

`14.7 * 333.55=324* 4.186* \Delta T`

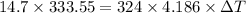
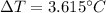`\Delta T=3.615^(\circ)C` is the drop in the water temperature.