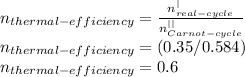Answer:
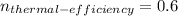
Step-by-step explanation:
Given data
Temperature T1=270K
Temperature T2=650K
Work=4.3 kJ
Heat rejects=8.0 kJ
To find
Thermal efficiency
Solution
The thermal efficiency is given as:
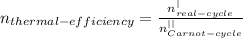
For Carnot cycle efficiency
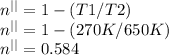
For real cycle efficiency
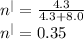
So the thermal efficiency is: